 PROPERTIES OF LOGARITHMS
PROPERTIES OF LOGARITHMS

SOLVING LOGARITHMIC EQUATIONS
1. To solve a logarithmic equation, rewrite the equation in exponential form and solve for the variable.
Example 5: Consider the function
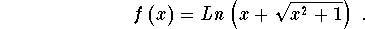
Find the inverse 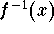 .
.
Solution:
-
- Step 1: From the graph, we determine that the function f(x) is one-to-one and therefore has a unique inverse. How can you tell from the graph that a function is one-to-one? Use the horizontal line test. Mentally run a horizontal line over the graph. If at any time, the line touches the graph in more than one place, it is not a one-to-one function.
-
- Step 2: Since f(x) is a one-to-one function, we know that the inverse exists. The inverse of a logarithmic function is an exponential function.
-
- Step 3: We know that the domain and range of f(x) are equal to the range and domain of
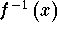 . From the graph of f(x), the domain is the set of all real numbers, and the range is the set of all positive real numbers.
. From the graph of f(x), the domain is the set of all real numbers, and the range is the set of all positive real numbers.
-
- Step 4: We know that the composition of a function with its inverse will yield x or

-
- Step 5: Find f(inverse):

-
- Step 6: Convert the equation to an exponential equation:
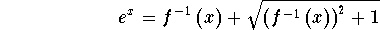
-
- Step 7: Isolate the
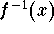 term using steps 8 through 13.
term using steps 8 through 13.
-
- Step 8: Subtract
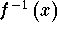 from both sides of the above equation:
from both sides of the above equation:
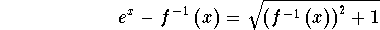
-
- Step 9: Square both sides of the above equation:
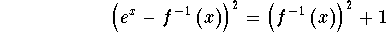
-
- Step 10: Expand the left side of the above equation:
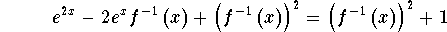
-
- Step 11: Subtract
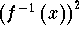 from both sides of the above equation:
from both sides of the above equation:
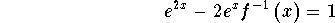
-
- Step 12: Subtract
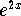 from both sides of the above equation:
from both sides of the above equation:
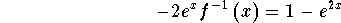
-
- Step 13: Divide both sides of the above equation by
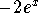 to get
to get
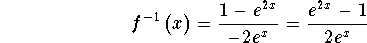
Check: You can check the problem by graphing the function f(x), graphing its inverse, and graphing the line y = x. If the graphs of the function and its inverse are symmetric to the line y = x, you have correctly found the inverse.
You can also check your answer by finding a point on the original graph, say (a, b), and determine whether the point (b,a) is on the graph of the inverse. This is not a perfect check, but it will alert you of a wrong
answer.
For example,
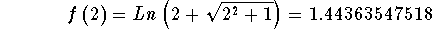
indicates that the point  is a point on the graph of f(x).
is a point on the graph of f(x).
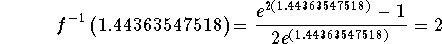
indicates that the point 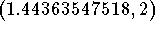 is located on the graph of
is located on the graph of 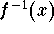 .
.
If you would like to review another example, click on Example.

[Back to Rules of Logarithms]
[Back to Exponential Functions]
[Algebra]
[Trigonometry]
[Complex
Variables]
 S.O.S MATHematics home page
S.O.S MATHematics home page
Do you need more help? Please post your question on our
S.O.S. Mathematics CyberBoard.

Author: Nancy
Marcus
Copyright © 1999-2024 MathMedics, LLC. All rights reserved.
Contact us
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA
users online during the last hour
 PROPERTIES OF LOGARITHMS
PROPERTIES OF LOGARITHMS PROPERTIES OF LOGARITHMS
PROPERTIES OF LOGARITHMS
![]()
![]() .
.![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() is a point on the graph of f(x).
is a point on the graph of f(x).
![]()
![]() is located on the graph of
is located on the graph of ![]() .
.
 S.O.S MATHematics home page
S.O.S MATHematics home page