
 | APPLICATIONS OF EXPONENTIAL |
| AND | |
| LOGARITHMIC FUNCTIONS |

EARTHQUAKE WORD PROBLEMS:
As with any word problem, the trick is convert a narrative statement
or question to a mathematical statement.
Before we start, let's talk about earthquakes and how we measure their
intensity.
In 1935 Charles Richter defined the magnitude of an earthquake to be
where I is the intensity of the earthquake (measured by the amplitude of a
seismograph reading taken 100 km from the epicenter of the earthquake) and S
is the intensity of a ''standard earthquake'' (whose amplitude is 1 micron =10-4 cm).
The magnitude of a standard earthquake is
Richter studied many earthquakes that occurred between 1900 and 1950. The
largest had magnitude of 8.9 on the Richter scale, and the smallest had
magnitude 0. This corresponds to a ratio of intensities of 800,000,000, so
the Richter scale provides more manageable numbers to work with.
Each number increase on the Richter scale indicates an intensity ten times
stronger. For example, an earthquake of magnitude 6 is ten times stronger
than an earthquake of magnitude 5. An earthquake of magnitude 7 is
![]() times strong than an earthquake of magnitude 5. An earthquake of
magnitude 8 is
times strong than an earthquake of magnitude 5. An earthquake of
magnitude 8 is
![]() times stronger than an earthquake
of magnitude 5.
times stronger than an earthquake
of magnitude 5.
Do you need more help? Please post your question on our
S.O.S. Mathematics CyberBoard.
Problem 2:
Answer:
Solution: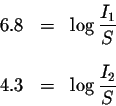
Solve for
![]() .
.
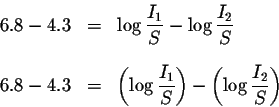
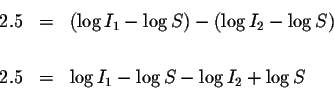
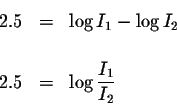
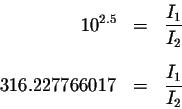
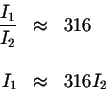
The intensity of the larger earthquake was earthquake as 316 times as
intense as the smaller earthquake.
Let's check our answer.
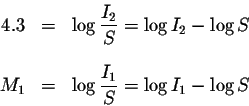
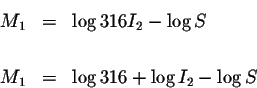
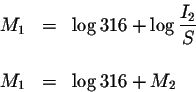
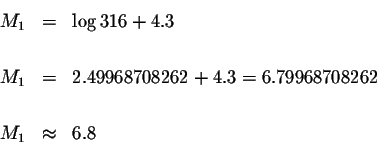
The check will not be exact because we rounded the answer. However, it is
close enough.
If you would like to review problem 2, click on problem.
If you would like to go back to the table of contents, click on
contents.

 S.O.S MATHematics home page
S.O.S MATHematics home page 
Contact us
Math Medics, LLC. - P.O. Box 12395 - El Paso TX 79913 - USA
users online during the last hour